Neuroscience
A couple of posts ago I raised the distinction between prediction and prospective control. I was trying to make the point that, if you are coupled to the right information, you don't need to be mentally simulating what's happening so you can run this simulation ahead and predict what's coming up. Prediction of this sort is invoked by representational cognitive scientists to cope with things like delays in the nervous system (e.g. Changizi's 'perceiving-the-present' framework). It's a risky business (if you make a mistake, you are suddenly controlling your behaviour according to an incorrect guess), and the better solution is prospective control. This is when you couple your behaviour to information in the world that doesn't tell you about the future, but that, if you use it for a while, will get you where you want to go.
People requested some more on this topic, and so here we go. People also requested something other than the outfielder problem, but I am going to start with this problem because it is still the best example, and I'll get into some more as we go to demonstrate it's not just baseball where this works.
The task
The first step in answering such a perception-action question is a task analysis. What, precisely, is happening in the task and what are the optical consequences of what's happening? In baseball, outfielders are often required to catch fly balls. These are balls hit high in the air, and the outfielder has to move from where they are to where the ball will land in the time it takes the ball to get there from the bat. The ball is typically moving pretty quickly, and is a long way away from the fielder, so it is very small. This last point means some types of time-to-contact information (like tau) aren't available, because the variation in the apparent size of the ball with changes in distance are below threshold. You can, however, learn to catch fly balls pretty reliably; the question is how.
The critical feature of a fly ball is that it moves through the world along a parabola; it is an example of simple projectile motion.
The distance something travels under projectile motion depends on the angle and velocity at the start, the object mass and size and air density, drag and gravity.
Potential solutions
1. Prediction
The prediction solution suggests you take the initial angle and velocity and use those to predict the parabolic path, assuming constant ball size and mass (fair, given it's a baseball) and constant air density, drag and gravity (less fair; drag varies non-linearly with things like spin and wind). If you have access the parabola, you simply read off where the ball will intercept the ground and go there to wait. However, even though you can estimate the ball's behaviour fairly well with some sensible assumptions about drag, etc (Saxberg, 1987a), people don't seem to use this strategy at all (Saxberg, 1987b).
2. Prospective control: Optical Acceleration Cancellation (OAC)
If people aren't acting on predictions generated by an internal simulation of the physics, they must be coordinating their actions using information available during the flight of the ball. The ball is too far away for information such as 'looming' to be of any use. However, there are certain optical consequences when the fielder moves relative to something travelling along a parabola that enable the fielder to refine that movement so as to intercept that object.
If you are aligned with the path of the ball (i.e. it is heading right for you) then the ball will appear to move upwards, slowing down until it reaches it's peak height and then speeding back up as it falls. In other words, the optical projection of the ball is accelerating. If you then move so as to cancel this acceleration, and make it appear that the optical projection of the ball is moving at a constant velocity, your movement will bring you to the right place at the right time to intercept the ball. If you are moving and the ball still appears to be accelerating, you must speed up in order to intercept it; likewise, if the ball is slowing down, you are running too fast and will overshoot. If you cannot move fast enough to cancel the optical acceleration then you will not catch the ball and you should prepare to catch the ball on the bounce.
This strategy has some appeal; it is an intrinsically perception-action strategy (you are required to move so as to produce a specific spatial-temporal pattern in the optics), there is continuous information about whether you are moving correctly, and you end up doing the right thing without ever knowing where the ball is going to land until you intercept it. However, while there is some support for it (most recently Fink, Foo & Warren, 2009) there are some problems. The first is that the evidence suggests people are not sufficiently sensitive enough to optical accelerations to be able to implement the strategy. Second, the geometry that produces the solution only works properly when your path and that of the ball are aligned; in baseball, this is often not the case. Third, as Figure 2 shows, you actually have to cancel out the acceleration of the ball along the slanted projection on the left; this means you have to cancel the acceleration of tan(α), and not α per se (where α is the angle between the ground and the ball as seend from your vantage point). Like tau, OAC is sound in principle, but the lawful relation between the world and the optics simply doesn't range over a suitably wide scope to allow stable behaviour.
3. Prospective control: Linear Optical Trajectory (LOT)
OAC is on the right track, but isn't good enough to support the observed behaviour (namely outfielders catching all kinds of fly balls). McBeath et al (1995) proposed a different strategy that shares the important features of OAC (continuously available information that allows you to control your behaviour with respect to your goal); this strategy is to move so as to make the ball appear to move, not along a parabola, but along a straight line. This strategy is called Linear Optical Trajectory, or LOT (Figure 3)
LOT is a 2-dimensional strategy. The outfielder's job is to move and vary both α (the vertical angle to the ball) and β (the angle along the ground) so as to make the ball appear to follow a straight line path. Varying both angles allows you to intercept fly balls when you aren't aligned to the ball's flight path and have to come in from the side. Instead of optical acceleration, the fielder needs to detect when the flight of the ball is optically curved, and they work to null this curvature. People are much more sensitive to this type of information, and it is an indirect way to detect optical acceleration (hence it works to intercept the ball).
Which strategy do people use?
It's looking good for LOT, but it is always an empirical question what information is being used, especially when there are alternatives (Gibson, 1979). The strategies entail different paths to the same location: prediction and OAC suggest that you will run along a straight path at constant speed; LOT suggests you will accelerate then decelerate along a curved path. Prediction also suggests you will simply run to a given location, whereas the prospective strategies suggest you will move according to the information, not the end location.
McBeath et al (1995) collected some simple data from 2 participants; they tracked the fielder's path and also recorded with a camera the optical trajectory that results from these paths. The paths were curved and entailed accelerations, while the optical trajectories were linear, on 75% of the trials. This suggests outfielders typically rely on LOT. McBeath et al also suggested that the fact skilled baseball players often run into walls and catch on the move, rather than rushing ahead of the ball, also work against the predictive strategy.
It's not yet over for OAC (see Fink et al, 2009); and people continue to run experiments showing that either LOT or OAC dominate in varying conditions. However, all the data support the idea that one of these prospective methods is being used, and prediction of any kind is simply not being made.
Summary
The reason I use the outfielder problem is that it is a complex, real world task that is amenable to the kind of detailed optical analysis required to identify potential sources of visual information for prospective control. That analysis has revealed at least two potential perception-action strategies in which people move so as to make the optic array contain a particular state of affairs, i.e. information. These strategies can be distinguished on the basis of the paths they predict people take (McBeath et al, 1995) and how those paths respond to certain perturbations (Fink et al, 2009). Even dogs can use these strategies (Shaffer et al, 2004), and demonstrating that non-human animals solve a task the same way we do is sometimes a useful way to help rule out the kind of complex internal representations of physics psychology thinks humans, but only humans, have. The outfielder problem in particular, and work on prospective control in general, shows how complex behaviour that looks like it requires a complex mental life can emerge by the operation of a simple rule over time (and Eric's discussing these topics with respect to Louise Barrett's book 'Beyond the Brain' on his blog).
References
Fink, P., Foo, P., & Warren, W. (2009). Catching fly balls in virtual reality: A critical test of the outfielder problem Journal of Vision, 9 (13), 14-14 DOI: 10.1167/9.13.14 Download
McBeath MK, Shaffer DM, & Kaiser MK (1995). How baseball outfielders determine where to run to
catch fly balls. Science (New York, N.Y.), 268 (5210), 569-73 PMID: 7725104 Download
Saxberg, B. V. H. (1987a). Projected free fall trajectories I: Theory and simulation. Biological Cybernetics, 56, 159-175. Download
Saxberg, B. V. H. (1987a). Projected free fall trajectories I: Human experiments. Biological Cybernetics, 56, 177-184. Download
Shaffer, D. M., Krauchunas, S. M., Eddy, M. & McBeath, M. K. (2004). How dogs navigate to catch Frisbees. Psychological Science, 15(7), 437-441. DOI Download
- Magic Trick Fools Us But Not Our Eyes
The magician throws the ball twice into the air and catches it, then he throws it a third time and it vanishes! Of course, he’s really secreted the ball in the palm of his hand, so why do so many observers believe they’ve seen the ball vanish mid-flight?...
- Affordance-based Control (fajen 2005, 2007)
The most commonly studied tasks in the ecological approach involve the perceptual control of actions such as interception and steering. These models all involve perceiving some variable and moving so as to null the discrepancy between a current value...
- A Field Spotter's Guide To Embodied Cognition
I've spent quite a bit of time lately on the blog and Twitter talking about what embodied cognition is not. For example, it's not about moving through time (Miles et al, 2010), and it's not about leaning to the left (Eerland, Guadalupe &...
- Embodied Cognition Is Not What You Think It Is
The most exciting thing in cognitive science right now is the theory that cognition is embodied. It is, in fact one of the things interested lay people know about cognitive science, thanks in part to a lot of high profile experiments that claim to show...
- Reading Group - Gibson (1979) Chapter 5 Part 2
Chapter 5 Part 2 In this part of Chapter 5, Gibson talks about how various relations between objects can be specified by the ambient optic array. This is important because image-based theories assume that we have to make a lot of inferences to detect,...
Neuroscience
Prospective Control I: The Outfielder Problem
A couple of posts ago I raised the distinction between prediction and prospective control. I was trying to make the point that, if you are coupled to the right information, you don't need to be mentally simulating what's happening so you can run this simulation ahead and predict what's coming up. Prediction of this sort is invoked by representational cognitive scientists to cope with things like delays in the nervous system (e.g. Changizi's 'perceiving-the-present' framework). It's a risky business (if you make a mistake, you are suddenly controlling your behaviour according to an incorrect guess), and the better solution is prospective control. This is when you couple your behaviour to information in the world that doesn't tell you about the future, but that, if you use it for a while, will get you where you want to go.
People requested some more on this topic, and so here we go. People also requested something other than the outfielder problem, but I am going to start with this problem because it is still the best example, and I'll get into some more as we go to demonstrate it's not just baseball where this works.
The task
The first step in answering such a perception-action question is a task analysis. What, precisely, is happening in the task and what are the optical consequences of what's happening? In baseball, outfielders are often required to catch fly balls. These are balls hit high in the air, and the outfielder has to move from where they are to where the ball will land in the time it takes the ball to get there from the bat. The ball is typically moving pretty quickly, and is a long way away from the fielder, so it is very small. This last point means some types of time-to-contact information (like tau) aren't available, because the variation in the apparent size of the ball with changes in distance are below threshold. You can, however, learn to catch fly balls pretty reliably; the question is how.
The critical feature of a fly ball is that it moves through the world along a parabola; it is an example of simple projectile motion.
 |
| Figure 1. Simple projectile motion follows a parabola |
Potential solutions
1. Prediction
The prediction solution suggests you take the initial angle and velocity and use those to predict the parabolic path, assuming constant ball size and mass (fair, given it's a baseball) and constant air density, drag and gravity (less fair; drag varies non-linearly with things like spin and wind). If you have access the parabola, you simply read off where the ball will intercept the ground and go there to wait. However, even though you can estimate the ball's behaviour fairly well with some sensible assumptions about drag, etc (Saxberg, 1987a), people don't seem to use this strategy at all (Saxberg, 1987b).
2. Prospective control: Optical Acceleration Cancellation (OAC)
If people aren't acting on predictions generated by an internal simulation of the physics, they must be coordinating their actions using information available during the flight of the ball. The ball is too far away for information such as 'looming' to be of any use. However, there are certain optical consequences when the fielder moves relative to something travelling along a parabola that enable the fielder to refine that movement so as to intercept that object.
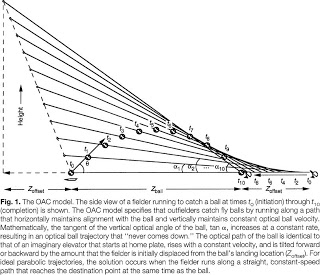 |
| Figure 2. Optical Acceleration Cancellation (OAC). From McBeath et al, 1995 |
This strategy has some appeal; it is an intrinsically perception-action strategy (you are required to move so as to produce a specific spatial-temporal pattern in the optics), there is continuous information about whether you are moving correctly, and you end up doing the right thing without ever knowing where the ball is going to land until you intercept it. However, while there is some support for it (most recently Fink, Foo & Warren, 2009) there are some problems. The first is that the evidence suggests people are not sufficiently sensitive enough to optical accelerations to be able to implement the strategy. Second, the geometry that produces the solution only works properly when your path and that of the ball are aligned; in baseball, this is often not the case. Third, as Figure 2 shows, you actually have to cancel out the acceleration of the ball along the slanted projection on the left; this means you have to cancel the acceleration of tan(α), and not α per se (where α is the angle between the ground and the ball as seend from your vantage point). Like tau, OAC is sound in principle, but the lawful relation between the world and the optics simply doesn't range over a suitably wide scope to allow stable behaviour.
3. Prospective control: Linear Optical Trajectory (LOT)
OAC is on the right track, but isn't good enough to support the observed behaviour (namely outfielders catching all kinds of fly balls). McBeath et al (1995) proposed a different strategy that shares the important features of OAC (continuously available information that allows you to control your behaviour with respect to your goal); this strategy is to move so as to make the ball appear to move, not along a parabola, but along a straight line. This strategy is called Linear Optical Trajectory, or LOT (Figure 3)
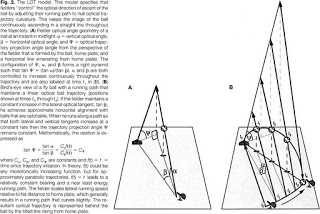 |
| Figure 3. Linear Optical Trajectory (LOT). From McBeath et al, 1995. |
Which strategy do people use?
It's looking good for LOT, but it is always an empirical question what information is being used, especially when there are alternatives (Gibson, 1979). The strategies entail different paths to the same location: prediction and OAC suggest that you will run along a straight path at constant speed; LOT suggests you will accelerate then decelerate along a curved path. Prediction also suggests you will simply run to a given location, whereas the prospective strategies suggest you will move according to the information, not the end location.
McBeath et al (1995) collected some simple data from 2 participants; they tracked the fielder's path and also recorded with a camera the optical trajectory that results from these paths. The paths were curved and entailed accelerations, while the optical trajectories were linear, on 75% of the trials. This suggests outfielders typically rely on LOT. McBeath et al also suggested that the fact skilled baseball players often run into walls and catch on the move, rather than rushing ahead of the ball, also work against the predictive strategy.
It's not yet over for OAC (see Fink et al, 2009); and people continue to run experiments showing that either LOT or OAC dominate in varying conditions. However, all the data support the idea that one of these prospective methods is being used, and prediction of any kind is simply not being made.
Summary
The reason I use the outfielder problem is that it is a complex, real world task that is amenable to the kind of detailed optical analysis required to identify potential sources of visual information for prospective control. That analysis has revealed at least two potential perception-action strategies in which people move so as to make the optic array contain a particular state of affairs, i.e. information. These strategies can be distinguished on the basis of the paths they predict people take (McBeath et al, 1995) and how those paths respond to certain perturbations (Fink et al, 2009). Even dogs can use these strategies (Shaffer et al, 2004), and demonstrating that non-human animals solve a task the same way we do is sometimes a useful way to help rule out the kind of complex internal representations of physics psychology thinks humans, but only humans, have. The outfielder problem in particular, and work on prospective control in general, shows how complex behaviour that looks like it requires a complex mental life can emerge by the operation of a simple rule over time (and Eric's discussing these topics with respect to Louise Barrett's book 'Beyond the Brain' on his blog).
References
Fink, P., Foo, P., & Warren, W. (2009). Catching fly balls in virtual reality: A critical test of the outfielder problem Journal of Vision, 9 (13), 14-14 DOI: 10.1167/9.13.14 Download
McBeath MK, Shaffer DM, & Kaiser MK (1995). How baseball outfielders determine where to run to
catch fly balls. Science (New York, N.Y.), 268 (5210), 569-73 PMID: 7725104 Download
Saxberg, B. V. H. (1987a). Projected free fall trajectories I: Theory and simulation. Biological Cybernetics, 56, 159-175. Download
Saxberg, B. V. H. (1987a). Projected free fall trajectories I: Human experiments. Biological Cybernetics, 56, 177-184. Download
Shaffer, D. M., Krauchunas, S. M., Eddy, M. & McBeath, M. K. (2004). How dogs navigate to catch Frisbees. Psychological Science, 15(7), 437-441. DOI Download
- Magic Trick Fools Us But Not Our Eyes
The magician throws the ball twice into the air and catches it, then he throws it a third time and it vanishes! Of course, he’s really secreted the ball in the palm of his hand, so why do so many observers believe they’ve seen the ball vanish mid-flight?...
- Affordance-based Control (fajen 2005, 2007)
The most commonly studied tasks in the ecological approach involve the perceptual control of actions such as interception and steering. These models all involve perceiving some variable and moving so as to null the discrepancy between a current value...
- A Field Spotter's Guide To Embodied Cognition
I've spent quite a bit of time lately on the blog and Twitter talking about what embodied cognition is not. For example, it's not about moving through time (Miles et al, 2010), and it's not about leaning to the left (Eerland, Guadalupe &...
- Embodied Cognition Is Not What You Think It Is
The most exciting thing in cognitive science right now is the theory that cognition is embodied. It is, in fact one of the things interested lay people know about cognitive science, thanks in part to a lot of high profile experiments that claim to show...
- Reading Group - Gibson (1979) Chapter 5 Part 2
Chapter 5 Part 2 In this part of Chapter 5, Gibson talks about how various relations between objects can be specified by the ambient optic array. This is important because image-based theories assume that we have to make a lot of inferences to detect,...
