Neuroscience
The Action Bits
The first part to be modelled is a rhythmically moving human limb (two of which will be coupled later). Two earlier perturbation studies (Kay, Kelso, Saltzman & Schöner, 1987; Kay, Saltzman & Kelso, 1991) had demonstrated that such a limb exhibited five key properties, all of which a model has to produce:
Bingham started with the so-called λ-model, a model of actual human limbs in which movements are generated by moving the equilibrium point of a damped mass-spring (see Feldman, 2010 for a recent overview). A mass spring is literally a mass bouncing on the end of a spring; a damped one includes friction and so, unless driven, it will eventually come to rest at it's equilibrium point. Feldman's basic suggestion was that a) human limbs are organised into synergies (Bernstein, 1967) best described as damped mass springs, and b) you could control these synergies easily by simply controlling the equilibrium point.
The basic form of a damped mass-spring is
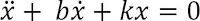 where x, x-dot and x-double dot are the state variables position and it's derivatives velocity and acceleration, and b and k are parameters (damping and stiffness, respectively). You keep such a spring moving by driving it (setting this equal to something other than 0). If the driver's value changes as a function of time, then you have a non-autonomous dynamic, which is no good. If, however, the driver's value is a function of the state variables (position and it's derivatives) only, then the spring drives itself - it is autonomous. With a suitable driver, this oscillator produces all the required characteristics from the list above.
where x, x-dot and x-double dot are the state variables position and it's derivatives velocity and acceleration, and b and k are parameters (damping and stiffness, respectively). You keep such a spring moving by driving it (setting this equal to something other than 0). If the driver's value changes as a function of time, then you have a non-autonomous dynamic, which is no good. If, however, the driver's value is a function of the state variables (position and it's derivatives) only, then the spring drives itself - it is autonomous. With a suitable driver, this oscillator produces all the required characteristics from the list above.
We now simply take two oscillators of this basic form, and couple them together via perceptual information.
The Perception Bits
This action system now needs to be controlled, and this is what perception is for. The driver on this system must be autonomous, specify relative phase and reflect the known characteristics of the perceptual information for relative phase:
You can control a single oscillator autonomously by driving it with it's own phase, because phase is simply the arctangent of velocity over position (i.e. it is composed of state variables):
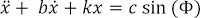 If you instead drive one oscillator with the phase of the other one, the two would now be coupled, but still autonomous. So far so good, but there's one final constraint: this coupling also varies as a function of relative phase (this is, after all, the key result from the coordination studies) and thus the drivers need to include a term for relative phase which is, itself, autonomous.
If you instead drive one oscillator with the phase of the other one, the two would now be coupled, but still autonomous. So far so good, but there's one final constraint: this coupling also varies as a function of relative phase (this is, after all, the key result from the coordination studies) and thus the drivers need to include a term for relative phase which is, itself, autonomous.
There is (indirect) evidence that this information term is the relative direction of motion; the HKB phenomena only emerge for parallel motion, i.e. when relative direction is uniquely defined (Bogaerts et al, 2003; Wilson et al, 2005; Wimmers et al, 1992). More recently we tested this more directly (Wilson & Bingham, 2008) but I will talk about that paper separately as it has a lot of important elements in it.
The current form of the model is therefore
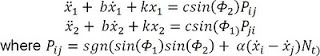 Pay close attention to the subscripts; oscillator 1 is driven by the perceived phase of oscillator 2, modifed by the relative direction term Ρ (rho). Ρ(i,j) is simply +/-1 depending on whether the two oscillators are moving in the same or opposite directions. The value is affected by a noise term proportional to the velocity difference (i.e. the ability to resolve relative phase depends on the relative speed).
Pay close attention to the subscripts; oscillator 1 is driven by the perceived phase of oscillator 2, modifed by the relative direction term Ρ (rho). Ρ(i,j) is simply +/-1 depending on whether the two oscillators are moving in the same or opposite directions. The value is affected by a noise term proportional to the velocity difference (i.e. the ability to resolve relative phase depends on the relative speed).
- Ecological Mechanisms And Models Of Mechanisms (#mechanismweek 4)
Mechanistic models are great, but so far cognitive science doesn't have any. We have functional models (of, for example, memory or categorisation) and dynamical models (of, for example, neural networks) but none of these can support the kind of explanations mechanistic...
- Perception, Action & Dynamical Systems
Over Easter I visited the Center of Functionally Integrative Neuroscience at Aarhus University in Denmark, courtesy of the Interacting Minds group. I gave a talk, got the tour, and met some of the faculty and students - some interesting opportunities...
- How To Build A Valid Measure Of Behaviour
One of the main problems facing psychology as a science is the issue of validity - what is the relationship between what you measured and what you are actually interested in? One of the things I like about studying movement is how straight-forward this...
- Coordination And The Haken-kelso-bunz Model
Other coordination posts are here. Now we have the basics out of the way, we can explore some of the key characteristics of coordinated rhythmic movements. Kelso's early experiments established a set of basic phenomena. Recall that, at the moment,...
- Coordinated Rhythmic Movement. - An Introduction To An Experimental Paradigm
I have some papers I wanted to talk about, but it occurred to me that they only make sense in a context. So I'm going to spend some time talking about the experimental task I use a lot, why it's interesting and what we can learn from it about...
Neuroscience
A Perception/Action Model of Coordination
Other coordination posts are here.
The role of perception in the dynamic leading to the HKB phenomena has been made clear by the work so far. But the exact form of this dynamic had yet to be modelled; the HKB model only consists of two cosine functions superimposed on each other to produce the two attractors at 0° and 180°, and the dynamic pattern hypothesis it embodies made predictions which did not held up empirically. It was now time to take an explicitly perception/action approach to modelling the task, which means it's finally time to turn to (my PhD advisor) Geoff Bingham's model (2001, 2004a, 2004b). This model is a fully perception/action model, and the modelling strategy Bingham lays out is, I think, a masterclass in how to go about building models of this kind.
Kelso was able to simply use cosine functions because he simply wished to describe the phenomena. Bingham had a more complicated problem; he needed to explicitly model the perceptual and action components, placing these in the correct organisation and coupling them together appropriately while still generating the key coordination phenomena. This model therefore operates under a long list of constraints.
The Action Bits
The first part to be modelled is a rhythmically moving human limb (two of which will be coupled later). Two earlier perturbation studies (Kay, Kelso, Saltzman & Schöner, 1987; Kay, Saltzman & Kelso, 1991) had demonstrated that such a limb exhibited five key properties, all of which a model has to produce:
- limit cycle stability: stability is a measure of the preferred state of a system, and you discover this by perturbing the system and seeing what it relaxes into doing. The attractors at 0° and 180° in the HKB model are examples of point attractors; a limit cycle is a periodic attractor, and so the system tends to relax into a periodic motion with some frequency and amplitude. Critically, this form of stability means that the underlying dynamic must be non-linear.
- phase resetting: phase is the measure of position within a cycle, and if you perturb a system you are effectively changing that position abruptly. If the system's position is a function of time, then the response to a perturbation at time t is for the system to go back to where the function specifies it must be at that time. If the phase resets itself (by returning to the limit cycle at a different point) then the dynamic is not being driven as a function of time. It is autonomous, i.e. being driven as a function of it's own behaviour.
- inverse frequency/amplitude relation: if you ask people to produce rhythmic movements and steadily increase their frequency, they will (if allowed) reduce the amplitude of their movements to achieve this.
- direct frequency/velocity relation: as frequency increased, so did the peak velocity. This unsurprising result is also a side effect of the mechanism for the frequency/amplitude relation (an increase in stiffness)
- rapid relaxation time, independent of frequency: the limb returned to the oscillation described by the limit cycle quickly and this time did not vary with frequency.
Bingham started with the so-called λ-model, a model of actual human limbs in which movements are generated by moving the equilibrium point of a damped mass-spring (see Feldman, 2010 for a recent overview). A mass spring is literally a mass bouncing on the end of a spring; a damped one includes friction and so, unless driven, it will eventually come to rest at it's equilibrium point. Feldman's basic suggestion was that a) human limbs are organised into synergies (Bernstein, 1967) best described as damped mass springs, and b) you could control these synergies easily by simply controlling the equilibrium point.
The basic form of a damped mass-spring is
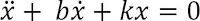
We now simply take two oscillators of this basic form, and couple them together via perceptual information.
The Perception Bits
This action system now needs to be controlled, and this is what perception is for. The driver on this system must be autonomous, specify relative phase and reflect the known characteristics of the perceptual information for relative phase:
- it must be in principle detectable by both vision and proprioception
- it must be more readily detected at 0° and 180° than anywhere else
- it must be composed of state variables only (for the overall dynamic to be autonomous)
You can control a single oscillator autonomously by driving it with it's own phase, because phase is simply the arctangent of velocity over position (i.e. it is composed of state variables):
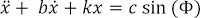
There is (indirect) evidence that this information term is the relative direction of motion; the HKB phenomena only emerge for parallel motion, i.e. when relative direction is uniquely defined (Bogaerts et al, 2003; Wilson et al, 2005; Wimmers et al, 1992). More recently we tested this more directly (Wilson & Bingham, 2008) but I will talk about that paper separately as it has a lot of important elements in it.
The current form of the model is therefore
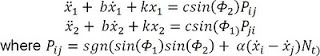
Summary
This model is the first (and currently the only) fully perception/action model of coordinated rhythmic movement. It explicitly models both perceptual and action components as having specific forms, and it places these components in a specific organisation with respect to each other. Critically, this model produces all the basic effects (0° and 180° the only stable states, 0° more stable than 180°, transition frequencies around 3-4Hz, plus limit cycle stability, autonomy and thus phase resetting* and the inverse frequency-amplitude relation). It instantiates a fully perception/action set of testable hypotheses about the identity of the information and the specific role it plays, and can be used to model both action and judgment experiments. It is, quite frankly, a master-class in how to go about building such a thing.
It is not over yet, however. The evidence for relative direction is compelling but indirect, and the model in this form cannot handle learning, say, 90°. My next post with discuss our perturbation study in which we identified the information for the visual perception of relative phase (Wilson & Bingham, 2008) and our recent empirical forays into learning which will constrain the future development of the model.
**************************
*Phase resetting has one interesting feature in human limbs movements. If you perturb an oscillator by slowing it down, the phase resets 'backwards' (i.e. it ends up behind where it would have been if left unperturbed); if you speed it up briefly, the phase resets 'forwards'. Human limbs, however, always rest 'forwards'; Kay et al hypothesised that this is due to the fact that actual limbs respond to perturbations by stiffening, a suggestion supported by the data and the modelling.
References
Bernstein, N. (1967). The coordination and regulation of movements. London: Pergamon Press.
Bingham, G.P. (2001). A perceptually driven dynamical model of rhythmic limb movement and bimanual coordination. Proceedings of the 23rd Annual Conference of the Cognitive Science Society, (pp. 75-79). Hillsdale, N.J., LEA Publishers. Download
Bingham, G. (2004). A Perceptually Driven Dynamical Model of Bimanual Rhythmic Movement (and Phase Perception) Ecological Psychology, 16 (1), 45-53 DOI: 10.1207/s15326969eco1601_6 (Download)
Bingham, G.P. (2004b). Another timing variable composed of state variables: Phase perception and phase driven oscillators. In H. Hecht & G.J.P. Savelsbergh (Eds.) Theories of Time-to-Contact. Boston: MIT Press. Download
Bogaerts, H., Buekers, M. J., Zaal, F. T., & Swinnen, S. P. (2003). When visuo-motor incongruence aids motor performance: the effect of perceiving motion structures during transformed visual feedback on bimanual coordination. Behavioural Brain Research, 138, 45-57. DOI
Feldman, A. G. (2010) Space and time in the context of equilibrium-point theory. Wiley Interdisciplinary Reviews: Cognitive Science. DOI
Kay, B. A., Kelso, J. A. S., Saltzman, E. L., & Schöner, G. (1987). Space-time behavior of single and bimanual rhythmical movements: Data and limit cycle model. Journal of Experimental Psychology: Human Perception and Performance, 13, 178–192. Download
Kay, B. A., Saltzman, E. L., & Kelso, J. A. S. (1991). Steady-state and perturbed rhythmical movements: A dynamical analysis. Journal of Experimental Psychology: Human Perception and Performance, 17(1), 183–197.DOI
Wilson, A. D., & Bingham, G. P. (2008). Identifying the information for the visual perception of relative phase. Perception & Psychophysics, 70(3), 465-476. Download
Wilson, A. D., Collins, D. R., & Bingham, G. P. (2005) Human movement coordination implicates relative direction as the information for relative phase. Experimental Brain Research, 165, 351-361. Download
Wimmers, R. H., Beek, P. J., & van Wieringen, P. C. W. (1992). Phase transitions in rhythmic tracking movements: A case of unilateral coupling. Human Movement Science, 11, 217-226. DOI
**************************
*Phase resetting has one interesting feature in human limbs movements. If you perturb an oscillator by slowing it down, the phase resets 'backwards' (i.e. it ends up behind where it would have been if left unperturbed); if you speed it up briefly, the phase resets 'forwards'. Human limbs, however, always rest 'forwards'; Kay et al hypothesised that this is due to the fact that actual limbs respond to perturbations by stiffening, a suggestion supported by the data and the modelling.
References
Bernstein, N. (1967). The coordination and regulation of movements. London: Pergamon Press.
Bingham, G.P. (2001). A perceptually driven dynamical model of rhythmic limb movement and bimanual coordination. Proceedings of the 23rd Annual Conference of the Cognitive Science Society, (pp. 75-79). Hillsdale, N.J., LEA Publishers. Download
Bingham, G. (2004). A Perceptually Driven Dynamical Model of Bimanual Rhythmic Movement (and Phase Perception) Ecological Psychology, 16 (1), 45-53 DOI: 10.1207/s15326969eco1601_6 (Download)
Bingham, G.P. (2004b). Another timing variable composed of state variables: Phase perception and phase driven oscillators. In H. Hecht & G.J.P. Savelsbergh (Eds.) Theories of Time-to-Contact. Boston: MIT Press. Download
Bogaerts, H., Buekers, M. J., Zaal, F. T., & Swinnen, S. P. (2003). When visuo-motor incongruence aids motor performance: the effect of perceiving motion structures during transformed visual feedback on bimanual coordination. Behavioural Brain Research, 138, 45-57. DOI
Feldman, A. G. (2010) Space and time in the context of equilibrium-point theory. Wiley Interdisciplinary Reviews: Cognitive Science. DOI
Kay, B. A., Kelso, J. A. S., Saltzman, E. L., & Schöner, G. (1987). Space-time behavior of single and bimanual rhythmical movements: Data and limit cycle model. Journal of Experimental Psychology: Human Perception and Performance, 13, 178–192. Download
Kay, B. A., Saltzman, E. L., & Kelso, J. A. S. (1991). Steady-state and perturbed rhythmical movements: A dynamical analysis. Journal of Experimental Psychology: Human Perception and Performance, 17(1), 183–197.DOI
Wilson, A. D., & Bingham, G. P. (2008). Identifying the information for the visual perception of relative phase. Perception & Psychophysics, 70(3), 465-476. Download
Wilson, A. D., Collins, D. R., & Bingham, G. P. (2005) Human movement coordination implicates relative direction as the information for relative phase. Experimental Brain Research, 165, 351-361. Download
Wimmers, R. H., Beek, P. J., & van Wieringen, P. C. W. (1992). Phase transitions in rhythmic tracking movements: A case of unilateral coupling. Human Movement Science, 11, 217-226. DOI
- Ecological Mechanisms And Models Of Mechanisms (#mechanismweek 4)
Mechanistic models are great, but so far cognitive science doesn't have any. We have functional models (of, for example, memory or categorisation) and dynamical models (of, for example, neural networks) but none of these can support the kind of explanations mechanistic...
- Perception, Action & Dynamical Systems
Over Easter I visited the Center of Functionally Integrative Neuroscience at Aarhus University in Denmark, courtesy of the Interacting Minds group. I gave a talk, got the tour, and met some of the faculty and students - some interesting opportunities...
- How To Build A Valid Measure Of Behaviour
One of the main problems facing psychology as a science is the issue of validity - what is the relationship between what you measured and what you are actually interested in? One of the things I like about studying movement is how straight-forward this...
- Coordination And The Haken-kelso-bunz Model
Other coordination posts are here. Now we have the basics out of the way, we can explore some of the key characteristics of coordinated rhythmic movements. Kelso's early experiments established a set of basic phenomena. Recall that, at the moment,...
- Coordinated Rhythmic Movement. - An Introduction To An Experimental Paradigm
I have some papers I wanted to talk about, but it occurred to me that they only make sense in a context. So I'm going to spend some time talking about the experimental task I use a lot, why it's interesting and what we can learn from it about...
